Pendulum is a very down-to-earth guy


So yesterday my author discussed about two very important systems of classical mechanics. Actually this guy seems to be obsessed with these two systems.

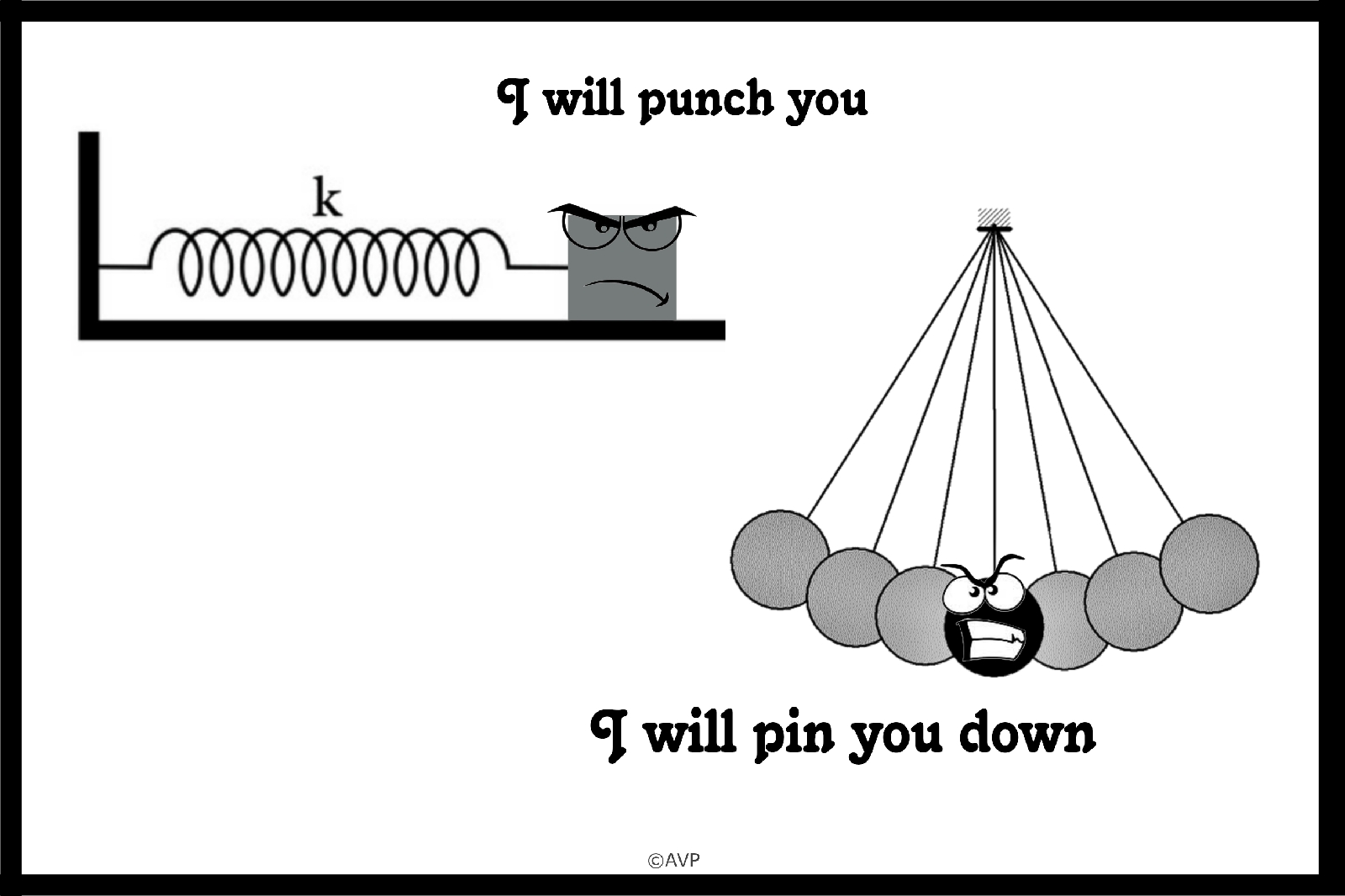
What he was talking about? A harmonic oscillator and a pendulum. An HO is different from a pendulum. That's because gravity plays no role in one but a major role in the other. Why is that so? Take out the spring from your pen and place it on the table. Hold it from behind so that it stays in position. Now push it inward. No need to hold on. Just release. What do you see? Did the spring bounce back? Ah yes, but does it bounce back to its original position? No. It moved beyond its position, right? And then we observe this to and fro motion. Well that won't last long since we have too many retarding forces in the real world. But that's not what we are worried about now. What is the role of gravity here? Did gravity play a role in bringing the spring back to its original position? No, it didn't.

But that's not the case with a pendulum. A pendulum is something whose whole existence and importance is due to gravity. Imagine you place a pendulum on a table, attach it to a thin rod, drag it to the left and release it. What do you see? We see the bob moving towards the right along with the rigid rod, right? No, you didn't. The bob remained where it was. Why was that so? Because the pendulum was not hanging but lying on a horizontal surface. The potential which should have driven it the original position doesn't exist. How will that potential come to life? We hang it upside down.

Hence the proof, the life of a pendulum revolves around gravity.


Comments

Post a Comment

Popular posts from this blog

How I wish mathematicians were normal people

As I was trying to understand my maths book, I came across the statement (below) and went on thinking and thinking 🧐. The statement: "Let R=Xî+Yĵ+Zk̂ be the position vector of any point in the tangent plane at P to the surface." Now.. What point? what tangent? Plane? And what surface? These random terms came flying from...vacuum? Uh, seriously very reader unfriendly. For some reason, academicians think it is ok to write unintelligible content.  Let me see how I would make my students understand this. "Girls and boys, close your eyes..and imagine a surface, a 3D surface. The shape? Just imagine a ball for convenience. We choose a point on it, dot it and name it P. Now extend this point (point P) in 2D and create a tangent plane. That is, imagine P spreading its wings in two directions so as to create a mat and hanging in air, on top of this ball, with just its belly button P touching the ball . N ow about this tangent plane: go left from the belly button and choose a p...
Read more

How operator p identified her child

Now we are wondering about our equation p̂ |Ψ⟩=p₁|Ψ₁⟩ Let's imagine |Ψ⟩ is the headmistress of Ramanujan public school. Now what about the symbols like | and ⟩? Well, they are the symbols of her authority as a headmistress. One is her large bun and the other is a large stick. One day, a parent named Ms. p̂ approaches the headmistress |Ψ⟩. Now why does p has a hat on her head. Let's say that is so since p "goes with an appeal to |Ψ⟩". So whoever has a request gets a hat on head 😉. What does she say to the headmistress? She says, "dear headmistress, since today is the last working day, we have planned a trip to Veegaland and will be leaving this evening. And so I want to pick my son." The school has hundreds of children and the headmistress |Ψ⟩ wouldn't know who is the child of Ms. p̂. So she asks, "what is his name?" Ms. p̂ tells her, his name is p₁. A clerk then goes to the class and picks a boy named p₁, the son of Ms. p̂. And this is how Ms....
Read more

Physics has the shape of water

Image
So yesterday my author told me how you change the shape of your model to fit your system and simplify your problem. Well this looks complex, I know (and it is, believe me). But maybe our police-thief story can simplify it.  (This is a story of how we make measurements using polar coordinates instead of Cartesian ones for certain systems.) The thread goes like this. You have a thief called Bob who is swinging in a single plane. Behind him are two policemen, Axe and Yay . The problem is, these two policemen go only two ways, forward and upward. Given their restriction, they have to work together to catch this guy. That means to catch one thief, we need two policemen, so if there are a 100 thieves, we need 200 policemen. Worried due to the inefficiency of the police of the society, the lawmaker appoints a committee. Their job is to find how to increase the efficiency of policemen. The committee studies the case and submits a report to the lawmaker. He analyses it and fin...
Read more